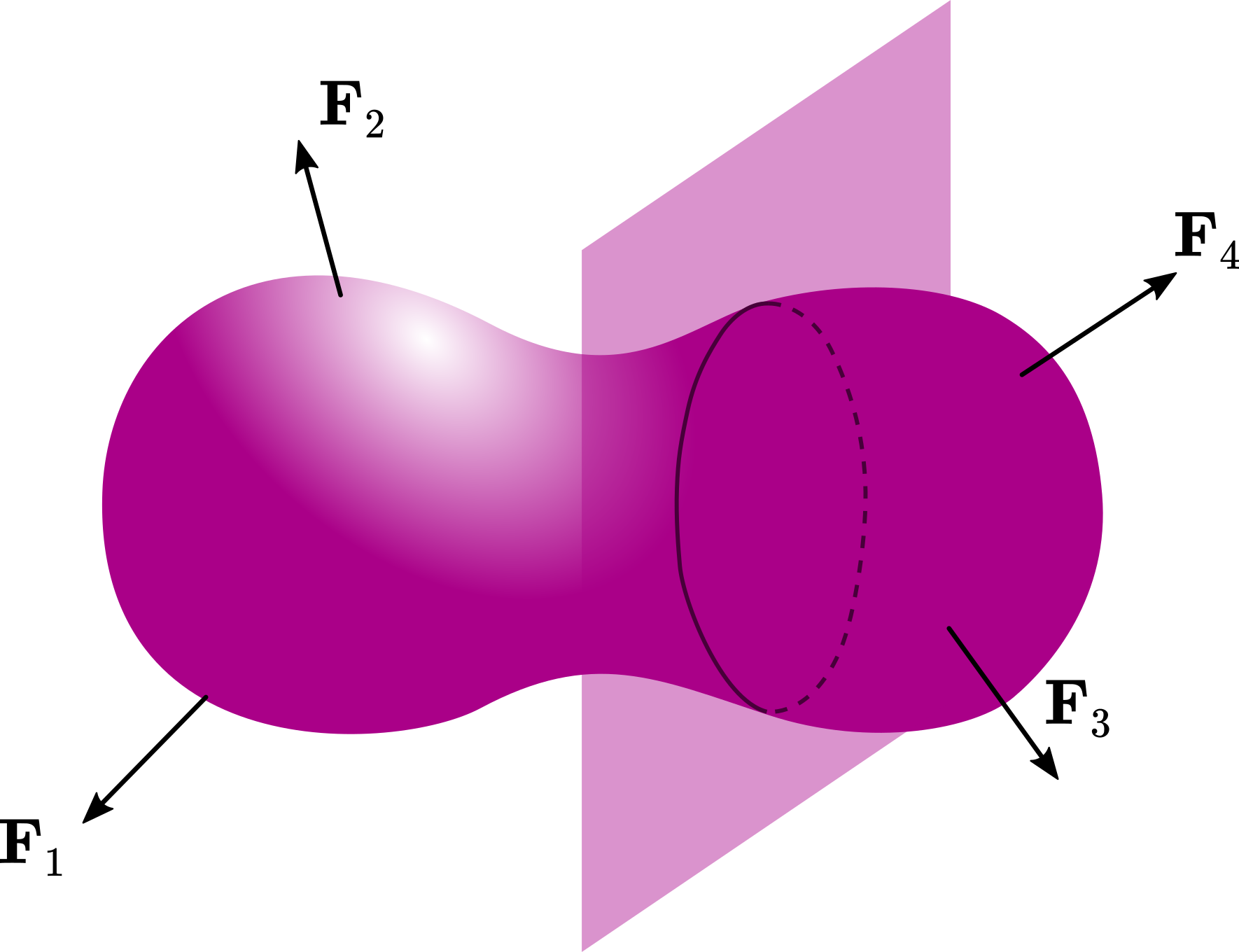
Consider a body subjected to external forces (Figure 1). The external forces tend to pull the body apart, crush it over, or in general deform it and produce rupture. The body resists against deformation and rupture because internal forces (i.e. actions and reactions between various particles) are developed within the body. The major concern of Mechanics of Materials is the determination of these internal forces that balance the effect of the externally applied forces.
 |
| Figure 1 |
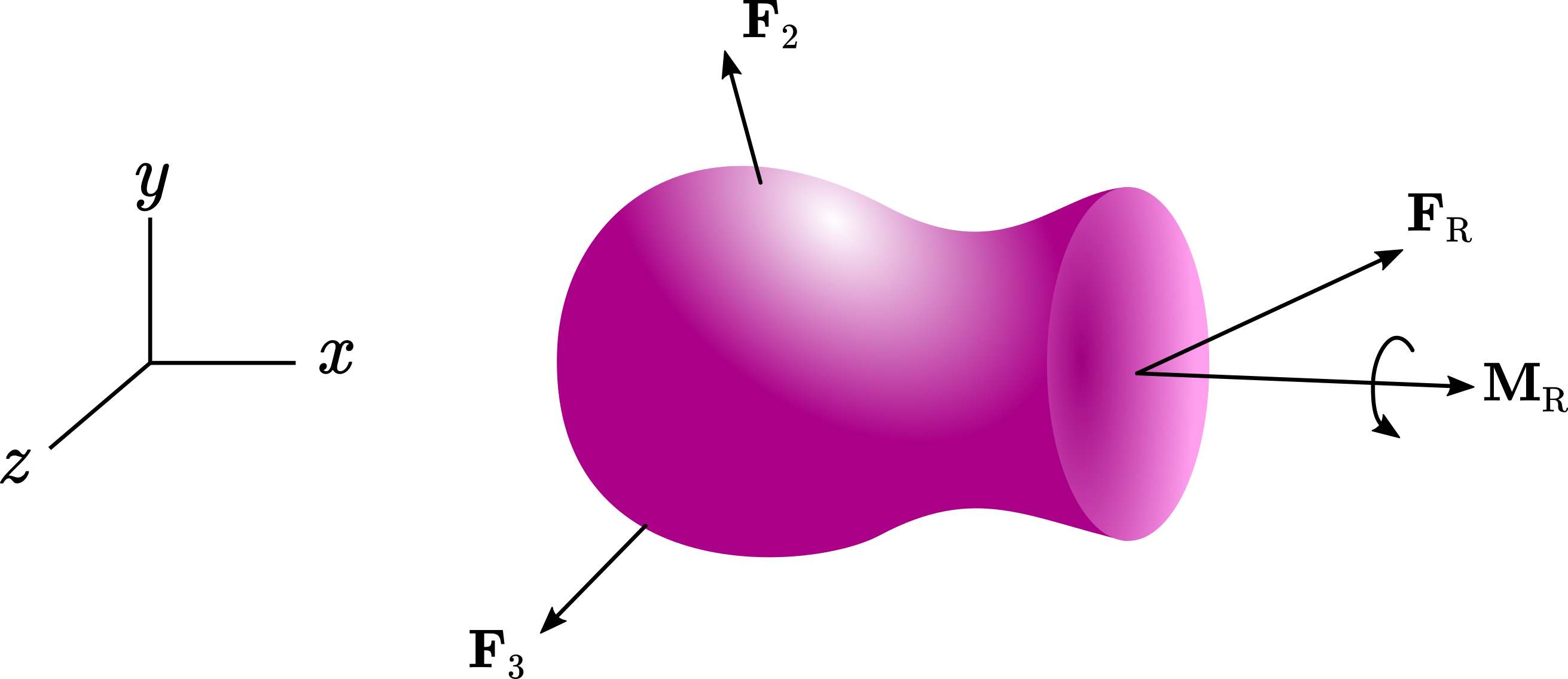
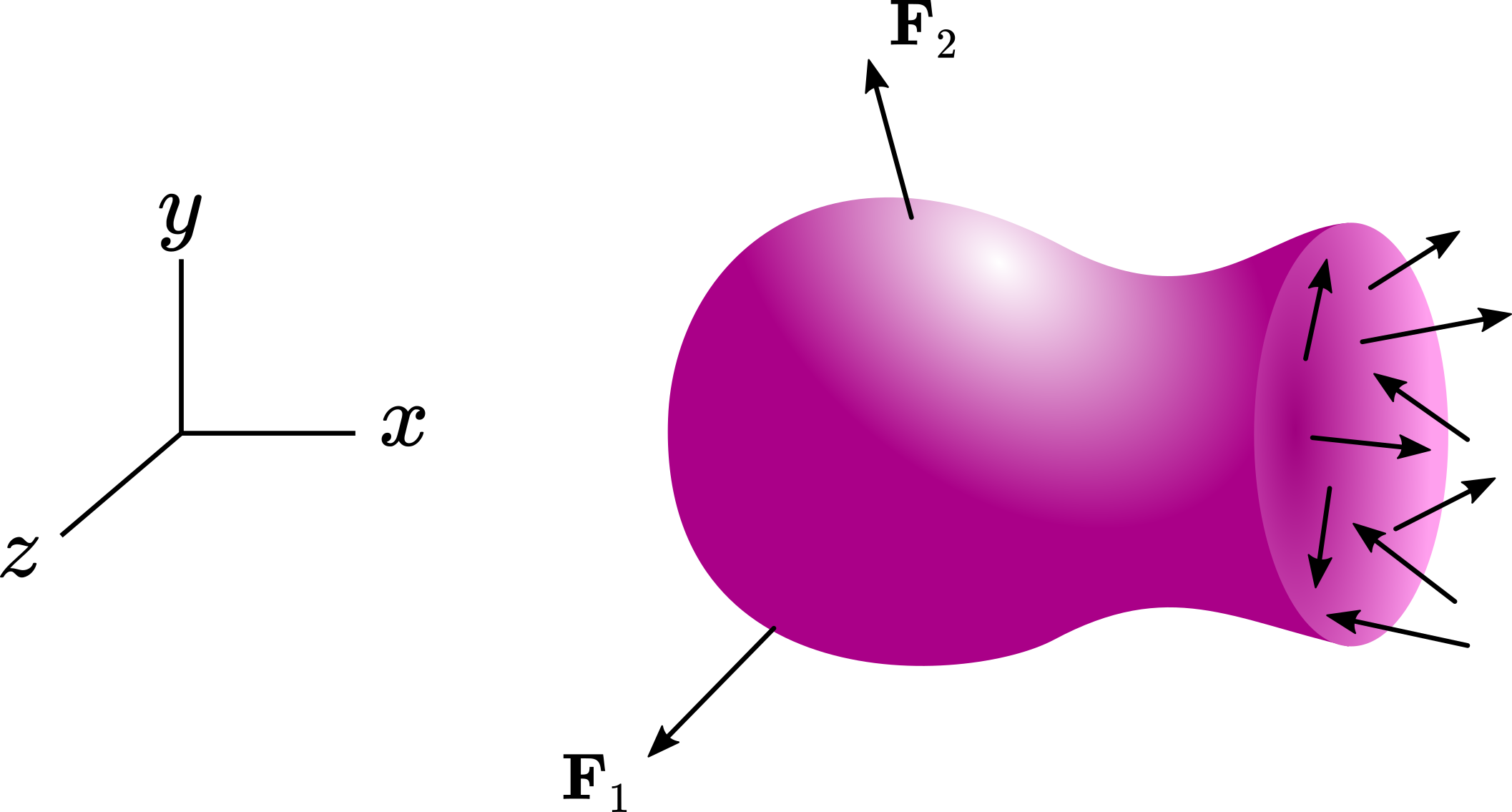
Assume the body is in static equilibrium. Let an arbitrary section be cut through the body, and completely separate it into two parts. Because the body is in equilibrium, each part of it must also be in equilibrium too and hence the external forces on one side of the arbitrary cut must be balanced by the internal forces at the cut. We can use statics to find the resultant force and the resultant couple moment at any position in the section (Figure 2a), but we wish to determine the distribution of the internal forces transmitted from one part of the body to the other through this plane (Figure 2b), and infinitely many force distributions yield the same resultant force and the resultant couple moment.
 |
 |
| (a) | (b) |
Figure 2(a,b)
In some special cases, the internal forces are the same at every single point of the cross-section. For example, if a prismatic bar is under tension or compression by external forces uniformly distributed over the ends, the internal forces are also uniformly distributed over any cross-section (Figure 3). However, in general, the internal forces vary in magnitude and direction from point to point (Figure 2b). In Mechanics of Materials, it is essential to determine the intensity of the internal forces (= the magnitude of force per area) at each point of the section because resistance to deformation depends on these intensities. The intensity of the internal forces is called stress. This chapter is devoted to the concept of stress.
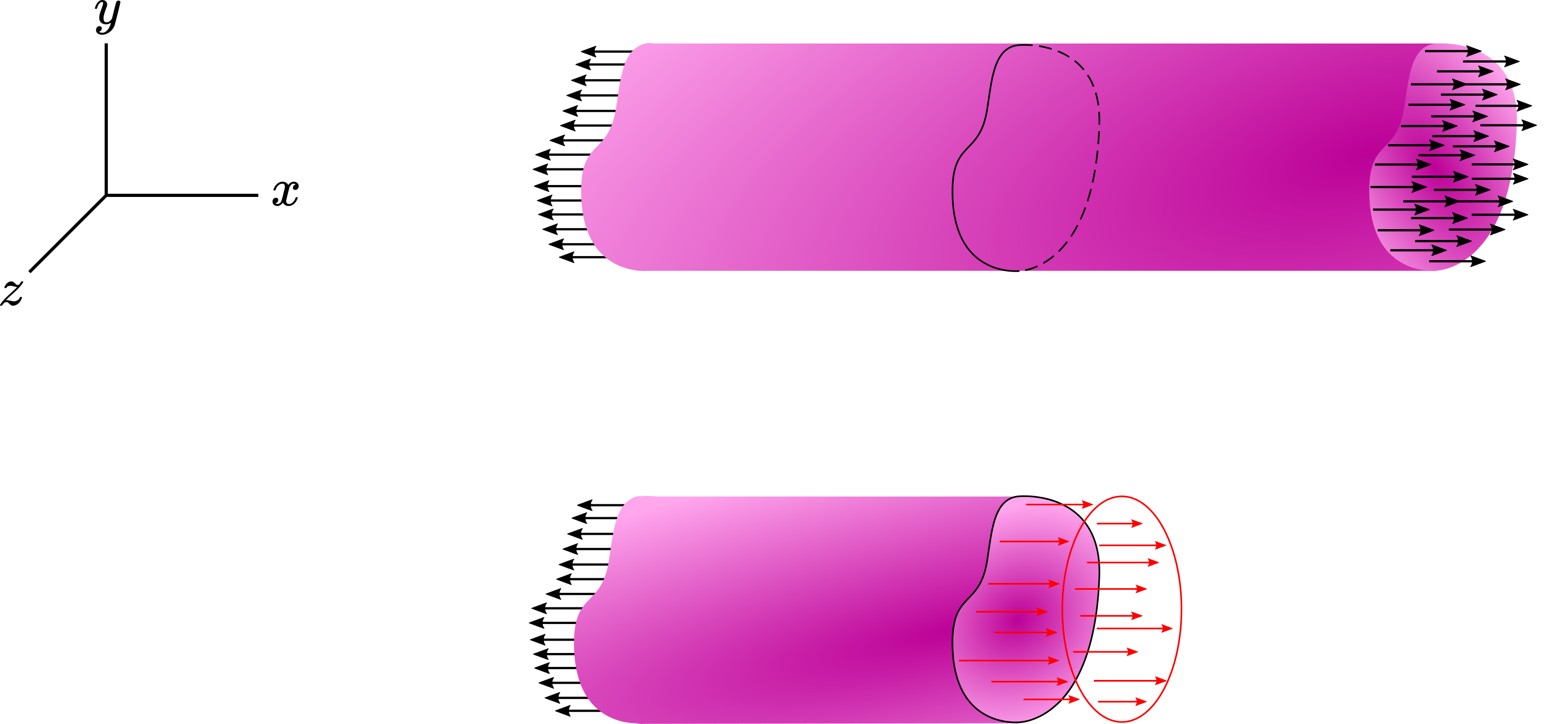 |
| Figure 3: The intensity of internal forces does not vary from point to point |
In this chapter, we learn:
- Stress State at a Point
- Average Normal Stress in Uniaxial Loading
- Average Shear Stress
- Bearing Stress in Connections
- Allowable Stress Design