A Quick Review of Pre-Calculus

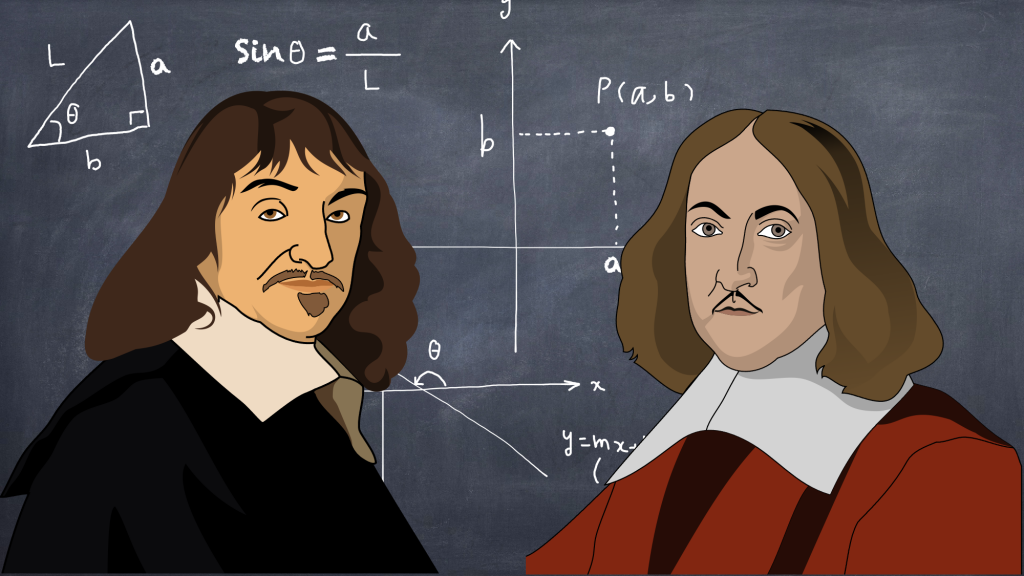
René Descartes (1595–1650), left, and Pierre de Fermat (1607–1665), right. Before the seventeenth century, geometry and algebra were two very distinct branches of mathematics. In 1637, Descartes made a huge impact on the development of mathematical knowledge by unifying these two branches of mathematics. His approach is now called analytic geometry. Independently of Descartes, Fermat discovered analytic geometry. Fermat developed a method for finding the tangent to curves and determining their maximum and minimum points that is equivalent to differential calculus. In a letter, Sir Isaac Newton stated that his own ideas about calculus were inspired by Fermat’s way of drawing tangents and applying it to abstract equations. Fermat is also called the founder of the modern number theory and co-founder of probability.

 

In this book, we quickly review what we will need to start learning calculus. Specifically, we review high-school algebra, analytic geometry, functions, elementary transcendental functions, and trigonometry. There are more than 100 fully solved examples.

 

Table of Contents