Previously, we defined the concept of a local maximum and a local minimum. We showed that if
Before we start, recall that if
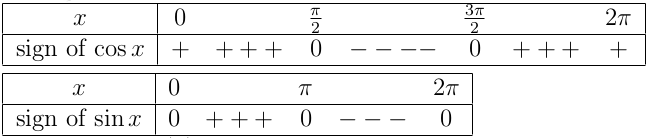
- As we move along the curve of a function from left to right, if the sign of the derivative changes from
- As we move along the curve from left to right, if the sign of the derivative changes from
- As we move along the curve from left to right, if the sign of the derivative on the left and on the right of a critical point is the same, the function has no local maximum or minimum at the critical point (see Figure 3).
More precisely, we have the following theorem.
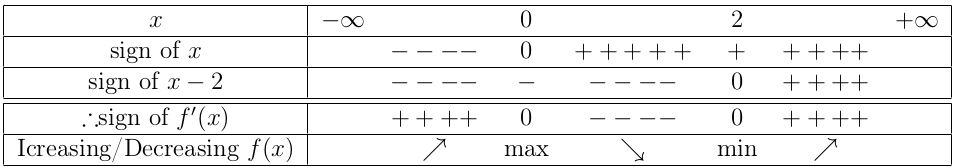
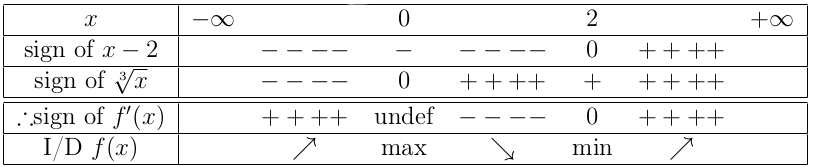
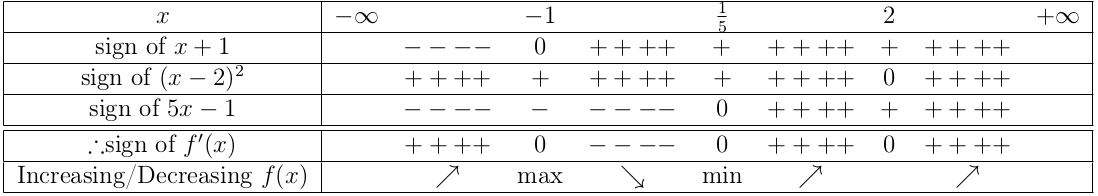
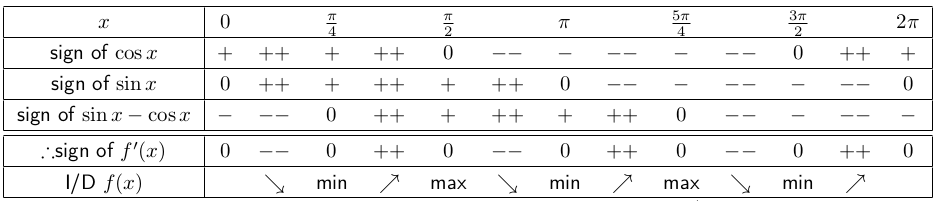
Theorem 1. (First Derivative Test) Assume
(a) If
(b) If
(c) If the sign of
- To remember the First Derivative Test, visualize
- Recall that the critical points are the only possible candidates for local extrema.
To determine the sign of the derivative at points near a particular critical point, an alternative method to the sign table is to first substitute a value of the variable a little less than the corresponding critical point into the derivative formula, and then one a little greater.